Finale

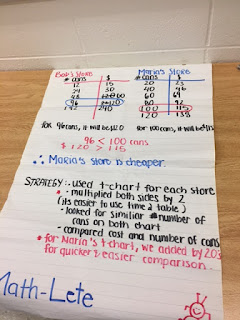
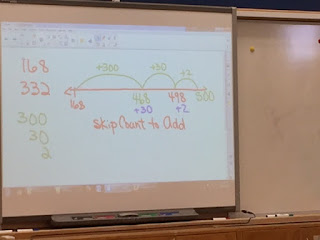
Before this class began, I couldn’t help but be nervous about teaching math. While I participated in our online math refresher course I was filled with so many emotions of doubt and anxiety. I hadn’t worked with many of these math concepts in years, so seeing them for the first time had me feeling uncomfortable, with the thought of teaching these concepts to a group of junior/intermediate students.  I found the refresher course to be beneficial but I wasn’t really fond of the way that it was structured. I think that it was a little crazy to have us jump in to math questions and concept right away, with our some sort of review before hand. I understand that they wanted to see how much prior math knowledge that each of us possessed, but I think that the way that this course was structured, many of us ended up feeling uncomfortable coming into math class.

Upon completing the math refresher course, I felt a little bit better about my math capabilities but I also wondered if I was the only one that felt this way about teaching math in general, and the refresher course. One the first day of class, I was immediately comforted by hearing that the majority of my colleges had similar feelings towards teaching math and the refresher course. It’s nice to know that you are not the only one this way. Pat also gave the class words of encouragement, hoping to change our opinions towards math to a positive math mindset and I must say, this helped me a great deal. I knew that changing my mindset towards math would not be easy but as the semester proceeded, working through our activity presentations, blogs, forums, and lesson plan, I can say that I now have a much more positive mindset towards math.

I think that one of the most important things that I have taken away from our math class is learning to have a growth mindset. In my experience as a teacher thus far, I have learned the importance of promoting a growth mindset within the classroom because many of the student that I will be teaching will have similar doubts and uncertainties about math, as I had as a student growing up. I have learned that one of the best ways to show students that they all have the ability to do mathematics is to provide them with manipulatives to help them gain deeper understanding of mathematical concepts. In class, Pat always did an amazing job of providing us with a variety of manipulatives such as blocks, shapes, toothpicks, and literally anything else you can think of to help us work through problems and build our confidence in math, to show us that we are all capable of doing this. This is something that I would definitely like to bring with me to my classroom.

Throughout the class, I feel as if I have grown so much as a teacher. I have learned many new strategies and concepts in this class.  I have learned how to use manipulatives effectively and how to incorporate them into my lesson plans and activities so that students can gain deeper understanding of mathematical concepts and idea.  I have learned about the many resources that are available to math teachers online and because of this, I have become more comfortable with planning lessons for my students in the future. Overall, I must say that in my experience in our math class has changed my perspective on math from a negative one to a positive one. I now have a growth mindset when it comes to math and that I something that I would definitely like to promote to my students.

Thanks, 

Kevin

Data and Probability

We have now finished up week 11 of math class and yet again, I am amazed at the various ways in which we can introduce mathematical topics and procedures to our students.  It is my goal to try and make math interesting and exciting, as I hope to change the negative perceptions about mathematics, to positive ones within my classroom.

In class this week, Pat brought in a jar filled with Oreos. I was immediately intrigued because I knew that we were about to engage in a fun activity that involved food.  For this activity we were supposed to use estimation to make an education guess on how many cookies were in the jar. We passed the jar around the class and people came up with their own unique strategies on estimating how many cookies were in the jar. Some people counted the cookies on one face-side of the jar and then used multiplication for the other sides of the cube to come up with an answer. Others, estimated how many Oreo’s came in a pack and tried to use that total to determine how many packs of Oreo’s Pat had added to the jar. Those are just some of the many strategies students used to come up with a number. Once everyone came up with their estimation, we placed our answers on a stem and leaf plot to visually see how our data was collected. Through this data, we could see the statistics of all of our numbers, such as the average number, the median, and the mode. I think that this activity was great because we use estimation all of the time in our daily lives and sometimes I think that we don’t even realize that we are doing it. I think that it’s important to show our students the many ways that we use estimation in our daily lives and give them useful examples like this.

Data collection is an important skill to possess. The textbook discusses the importance of organizing and sorting your data and creating appropriate questions when collecting your data, so that you gather your information in the best way. The image below shows the use of linking cubes to looks for patterns, make predictions, make comparisons, and make decisions. In class we have used linking cubes a lot to work through mathematical problems. I think that manipulatives such as linking cubes are a great way for students to engage in data collection because they are able to physically see and work with the different types of data that these cubes can represent. 

While browsing YouTube I found a fun and interesting video about Yoda and how he is teaching young Luke Skywalker to have a grown mindset. In the video Yoda tells Luke to “unlearn what you have learned”. Luke replies saying, “ Alright, ill give it a try” and Yoda says “ No! Try not! Do or do not! There is no try”. This scene is very relative to my experience in our class thus far. I feel like I have had to unlearn some of the “old” ways of math and I have had to have a growth mindset to tell myself I can do this!

Teamwork Makes The Dreamwork

As we get closer to our first teaching block I feel that it is necessary to reflect upon some of my experiences that have happened so far in our mathematics class. I personally feel that my thoughts and opinions of math have drastically changed from negative to positive and this is due to my experiences working with new fun and engaging concepts. This class has given me confidence by showing me a multitude of new ways to make math inclusive, fun and educational. It has also showed me the amount of resources that a available for teachers to improve the learning experiences in their classrooms.The text and technological resources that we have used in class have giving me much more confidence knowing that there is something that I can turn to for ideas and additional support.

This week, we began the class with another collaborated activity. We had coloured Popsicle sticks with numbers ranging from 1 o 6 and each of us picked a number to form a group of six.  This is a great technique because it helps establish groups with some choice but also allows for students to work with some new faces and get to know their classmates. I can definitely see myself using this strategy in my classroom. I think that this strategy works well to promote an inclusive environment in your classroom.

Once we were in our groups, we had a choice to select a station where different types of tasks were placed at each table. The first two stations that my group chose had a mathematical problem that involved toothpicks. Using toothpicks, we had to use the 5 descriptive cards to try and create the shape that the cards were describing.  This activity was somewhat challenging because you had to use the descriptive cards to create the shape. Even with the collaboration of your group members, it still provided a challenge.

The third station had a similar objective using descriptive cards to create a shape, except this time the cards were used to create a pattern. We used the manipulatives to create the pattern in the image posted below:

The activities in our class this week were excellent. It felt great to work with my classmates to try and solve these fun and interactive problems. I really do enjoy and appreciate the use of physical manipulatives and I believe that they are very beneficial to accelerate students learning. To conclude my blog post this week, I would like to say that I am actually kind of sad that class will be coming to an end. I wish that I could absorb more of the mathematical concepts and content before I enter my teaching block. With that being said, I am very grateful for everything that I have learned during my time in math class and I look forward to using all that I have learned in my teaching block to try and heighten my students learning experience and perhaps teach them things that I may have not had the opportunity to learn when I was in grade school.

The Life of Measurement

It is coming to the end of the term now and I can officially say that I have learned a lot during my time in Mathematics. I have had the opportunity to learn new and fun was of teaching math and that makes me very happy because I feel like my school experience in math was for the most part, taught right from the text book. I am looking forward to what the last few weeks of class will bring.

In class this week we focused on the topic of measurement.  I can recall that I was not particularly fond of this subject area when I was younger but I think that I failed to realize its relevance and importance in everyday life. We can use tools to measure length, area, perimeter, weight, volume, temperature, and even time in our everyday lives.

The textbook does a great example of providing students with everyday examples to help guide their understanding of measurement. I think this is extremely important for our students because often, If we can not relate mathematics to something useful in our lives, then we easily loose interest in the subject matter. A great example from the textbook is using your fingers to measure something.  Such as, “My finger is 1 cm wide, so this string must be 1,2,3,4,5 cm long”. Children often do this without even realizing that they are engaging in a mathematical process. Which, is why it is important for us as teachers to remind our students of the mathematical processes that they take part in on a regular basis.

I think that it is also important for us to stress the metric system within our classroom. I think that there is a major confusion with our students because they are often exposed to using the imperial system when we are told to us the metric in Canada.  It can be beneficial to be knowledgeable of both systems but why confuse our students when the mathematical curriculum is based on the metric system?

This week one of our classmates did their learning activity presentation using a technological example. Christian presented his information to the classroom using Kahoot! as a strategy to engage the students. I found this activity to be fun and it allowed for us to collaborate among our peers to work together and come up with solutions. As a teacher, I can see myself using an activity like this in my classroom as a minds on, to get my students thinking about the particular topic and engaged with their peers so that they can bounce ideas off of each other and work collaboratively to come up with different solutions to problems.

With a few weeks left of class, I am eager to use the strategies that I have learned thus far, during mathematics in my placement. I am now much more confident in my mathematical abilities then I was before this class and I look forward to showing what I have learned to my students

Shapes Are Fun!

This week’s math lesson focused on the tops of Geometry and Symmetry. To begin class, we began an activity where each group was given Halloween shapes and we were asked to find and discuss the similarities between each of our shapes. We came the conclusion that each table was given the same shape but each shape had different properties. This task was important because it helped us define what the term “similar” meant in relation to mathematics and geometry. Following this exercise we discussed symmetry and what it means for something to be symmetrical. We used examples and manipulatives to help us understand that, in order for something to be symmetrical it must have sides or halves that are the same. For example, If you split a square in half than both sides are symmetrical because both sides are the same.

I am becoming very fond of the idea of introducing mathematical concepts to students through story telling.  I think that it gives students a fresh perspective on mathematical topics that are relative to their experiences through their young and adolescent lives.  If students can relate to something, then it makes it so much easier for them to understand concepts and ideas. Mathematics is largely about relationship and if we have experienced something throughout our lives then we usually have a deeper understanding of concepts and problem solving.  The book called “ The Greedy Triangle” by Marilyn Burns introduces children to shapes and polygons.  This book is excellent for early learners of math and can be connected to the mathematics curriculum for geography, within many grade levels. As a teacher I would use this as a resource to extend my students knowledge of simplistic shapes and then carry on to discuss shapes beyond those mentioned in the book. Overall, I thought that this book was an excellent resource that could be used for many grade levels. 

In class this week, Courtney had a great geometry activity. She began her activity by introducing the class to symmetry using drawings on the board and folding paper shapes as a physical manipulative. The point of the introductory activity was to help refresh our minds on finding lines of symmetry. Afterwards, she had a secondary activity, where students went on a treasure hunt for Halloween candy. Students had to determine whether or not, shapes were symmetrical or asymmetrical and then determine their lines of symmetry. Once they identified these properties they had to fill them in on the activity sheet. The first group that completed the activity sheet correctly received the pirate’s treasure. I though that this was an excellent activity to introduce students to symmetry and working with 2-D and 3-d shapes. I really liked the assortment of the learning activities that we had in our class this week. As a teacher candidate, I can envision myself using many of these activities that we have seen thus far in our class because they have been extremely creative and have provided students with the opportunity to engage in exciting mathematical activities, where any student can participate with confidence. 

Week 7- Patterning and Algebra

I find it quite ironic. I really enjoy patterns but in the past I’ve never been fond of algebra. Even though, they are two strands of math that essentially complement each other, the thought of algebra always scared me because I was not very comfortable with algebraic equations. I also never realized the relationship between patterning and algebra and I cannot recall if both strands were taught together when I was in elementary school. Therefore, as I read the assigned chapter for this week, it was interesting to see the relationships between the two.

One of the most important things about Patterning and Algebra is learning to find and understand patterns. Patters can appear in many different forms such as, colours, shapes, and numbers and in math we have to possess the ability to find and determine the relationships within these patterns so that we can progress into a deeper knowledge into algebraic thinking. In class, we worked on an excellent algebraic matching exercise, which got our minds thinking about the relationships between patterns and algebra. We were given a set of cards and were asked to match cards that fit specific algebraic equations. For every equation, there had to be a matching graph card, t-chart card, and pattern block card. This exercise was a great way for students to recognize the relationships between the patterns and the algebraic equations. It also gave students the opportunity to visualize the many different ways that these patterns and equations could be solved. I think that providing students with activities and manipulatives such as these are great because they stimulate the student’s minds into thinking outside of the box to recognize that math problems can be solved in many different ways.

The textbook suggests that teachers sometimes overemphasize growing patterns over shrinking patterns. It extremely important for teachers to provide practice using all different types of patterning because down the line when students are asked to solve difficult algebraic equations, some students will not be able to recognize and determine the patterns because their teachers rushed through patterning in their early grade and did not give students the practice that they needed to develop their patterning and algebra skills in the future.

In class we also had the opportunity to use blocks as psychical manipulatives to see what increasing and decreasing patterns can look like in their different stages.  To do this, Pat had us use two different block colours to help us recognize the change in our patterns. For example, the picture shown below has a constant of 2 red blocks and an increase of 2 each time. Therefore, our equation for this growing pattern is (2s+2).  I really enjoyed using the coloured blocks as a physical manipulative. It really helped me to visualize everything that was involved throughout the pattern and the equation. I think that it is extremely important for teachers to use physical manipulatives because it allows students to construct their own ideas for mathematical processes and equations and it gives them the opportunity to explore different ways to communicate their understanding. 

Teaching strategies

This week in class we participated in a math congress. Mathematical communication and discussion is essential for learning mathematics because as we communicate we are able to reflect on, clarify, and expand our ideas. The math congress was a great exercise that began with the teacher assigning students to work in a group and work together on chat paper to create a solution to the problem that the teacher assigns. The poster should have ideas that are well thought and should present important strategies and concepts that were used to solve their problems. While the students are working away on their problems, it is a great time for the teacher to walk around the class and assess how the students are doing. In our Math congress in class Pat was walking around as we were working on our problems and she got a great idea of how everyone was doing in regard to their understanding of the problem and she was able to see the different mathematical strategies and the different ideas that each of our groups were working towards. I can definitely see myself using a math congress in my classroom because I think that it is extremely important to have discussions in math and if students are not comfortable discussing mathematics with the teacher during the lesson, this gives them the opportunity to discuss ideas and concepts with their peers and gives them the chance to collaborate critically.

As we finished up working on Joel’s Kitchen problem, we posted our solutions around the class and has a gallery walk. This is a unique strategy that has studnets move from station to station to view everyone else’s work. the gallery walk allows students to get into discussion about the problem in a mode of active engagement. It allowed us to see the many different strategies and methods that others took, which helped us gain a deeper understanding of Joel’s problem. I like this strategy a lot because it helps the students understand that there is more than one way to solve mathematical problems and this is beneficial because some individuals may not get how to do a mathematical problem one way, but they understand how to do it another. The gallery walk is also beneficial to teachers because it allows the teacher to monitor the classroom and assess how the students are collaboratively working to understand the mathematical concepts.

After the gallery walk, it is a good strategy to have students return to their original groups to discuss things that they noticed and to reflect on the overall process. Students can then decide if they would like to add things to their original problem and think about whether or not they would have approached the problem in the same way, with their newly acquired information. This gives students opportunities to come to final conclusion on what they saw and discussed and it also gives the teacher the chance to assess the overall classroom’s understanding of the problem and allows the teacher to provide feedback.

Satisfaction With Fractions Pt. 2

   We are approaching week 6 in Math class and this week we finished up working with fractions using division. We began the class with an exercise of Red Light, Green Light. This game had students engage in the activity where the goal of the game is to get from one end of the playing area to the other, as the teacher says “red light” to stop and “green light” to go. Afterwards, we worked with a problem that had examples of the student’s final placement in the game, which was represented by a fraction. For example: Kevin finished 8/9, Kelly finished 5/6, and Lianne ¾, etc. Students were then asked to organize the fractions in order. This activity was great because it was fun and interactive. It allowed students to participate, regardless of their math comprehension level and there were many different ways that students could approach this problem. As teachers, we must teach our students the big ideas about fractions. We have provide students with the basic techniques so that they are able to recognize what a fraction is. A fraction is formed by splitting a whole into any number of pieces of equal size. They should be able to recognize that factions and ratios are the same and that whole numbers are not fractions. A big part of learning fractions is recognizing and differentiating “what is” and “what is not” considered a fraction. Once students have these foundations, it makes it much easier for them to begin adding, subtracting, multiplying, and dividing fractions.

 In class we also had a word problem that revolved around a story where a man named Mr .Tan had a very important porcelain artifact. He accidentally dropped this artifact and it broke in seven pieces. He noticed that the pieces had broken in different shapes and sizes and wonder if he could put this artifact back together. As a class, we were given the task to help Mr. Tan put his artifact back together using similarly shaped tangram pieces. We had to find a way to put our seven pieces back together in a square as displayed in the image below. Then as a class we tried to estimate what faction of each shape, fit into the square.

 I really enjoyed this activity and thought that it was a great way for students to get involved with thinking about fractions. When I was a student I would have really appreciated working on a word problem like this because it was something that was fun, interactive, and included a mixture of storytelling and hands-on activity, where everyone can get involved. Also, working with tangrams is always appealing to students because they are fun and they allow students to visually see the size of the shapes, so that they can explore many different ways to repair their square artifact. Even if the student cannot repair their square using the tangrams, they can still see the size of the shapes and this could help them determine the potential fraction that each shape represents to make the entire square.

Satisfaction With Fractions

We have just finished week 4 in math class and things are still going very well. My practicum has given me the opportunity to see how students math proficiency is assesses, as the students in my practicum class have had math assessments on both days of my placement. This has been a good experience thus far because I have been able to look at some of the student’s tests and see how the teacher grades the assessment, according to the marking rubric. It has also given me the opportunity to see some of the different ways that students approach particular math questions. I found it very interesting that some students are very particular with showing their work and other do not do this but they still get the answers correct.  I struggle with this because I think it leaves teachers in a dilemma. If a student consistently gets his or her answers right but doesn’t show their work, do we mark it wrong? Do we give part marks? I know that showing your work is an extremely important thing to do in math but not every student will do this. We unfortunately do not live in a perfect world. So, do we suggest that if the student doesn’t show their work, they do not understand the material or the expectations? Is the student just lazy? That is some food for thought.

The only downside to my practicum thus far, is that I haven’t had the opportunity to see how my practicum teacher teaches the math material. Hopefully when we are back in our observation period I will have the opportunity to see my practicum teacher’s instructional methods before I begin my full-time placement in her class. Thankfully, our math class has been very informative and highly beneficial thus far, so I feel more confident about teaching math.

I really enjoyed our last class. We read the Hershey’s book on fractions. I think that this is an excellent resource for teachers to help their students work with and understand fractions. Having chocolate bars as a manipulative to work with fractions is genius. Students get to see visual representations so that they can understand how fractions work and get the chance to work through it with their peers and demonstrated their understanding. We also used other manipulatives such as the ones shown below, to work with fractions and see how many different manipulatives can be used to help students gain a deeper understanding of how to work with various types of fractions. The egg carton was another strategy used as a visual representation to increase students understanding on how fractions work. As we add and remove eggs to the carton, students can figure out that 6 eggs in a carton of 12 equals ½, ect.

 As teachers, it is important for us to give students visual representations and come up with questions that are open-ended. This gives the students the opportunity to engage in discussion about the problem with their peers and while this is happening, the teachers can assess the students understanding of the problems and provide them with feedback.

Alternative Methods for the Future

  We are now in week 3 of math class. What a ride it has been thus far. In class, we discussed the importance of encouraging teachers to use alternative algorithms. In math we use algorithms as a particular procedure or step to get our students to follow to preform calculations. Traditionally, teachers would show their students one way to calculate a formula or a math problem and then move on with the unit. The problem with this is that, not every student can relate to that particular way of teaching. Every student has a preferred way of learning and giving them one option to complete as task could be setting some students up for failure. Therefore, I believe that it is important to give students more variety and different ways to complete a math problem so that they have options and that way; they all have the opportunity to succeed in math. The curriculum has evolved since I was in elementary school and this new strategy of providing students with alternative algorithms is great. I wish that I could have had the opportunity to learn this way because I believe that I could have been a lot stronger in math then I currently am.

  In class this week we also looked at algorithms for adding and subtracting whole numbers. I was amazed to learn that there were actually additional methods of adding and subtracting numbers. Its funny because I was completely oblivious to the fact that basic math such as addition and subtraction could be taught in more ways than one and this is mostly due to the fact that growing up, I was only taught one way to formulate equations in school. In our class, we learned how to add and subtract using skip counting. I think that this alternative method of adding and subtracting is great because it not only gives students a different way to learn and understand the concepts of these processes but it also potentially provides students with a simpler way to calculate equations. For example, traditionally when subtracting whole numbers, we were taught to use borrowing. Many students have difficulty with the concept of borrowing and in my opinion it is not the best way of teaching subtraction and it is just one example of a method of teaching that has worked for some but will not work for everyone. The Image below displays an example of using skip counting to add: If a student has to add 168 to 332 they can place 168 on a line and turn 332 into 300 +30+2 when adding it to 168. Such as 168+300 =468 +30=498+2=500. See image below:

   As educators we should be committed to providing our students with alternative algorithms so that they don’t have a false belief that there is “ only one way” to Add, subtract, multiply, and divide. With math scores and proficiency plummeting across the country there is a need for educators to provide students with the proper tools for success so that they can flourish in the future. Here is an interesting alternative algorithm video :)

Welcome!

On the first day of class we introduced ourselves and talked about our previous experiences in Math, so I figured that I would start my welcome blog the same way. My name is Kevin Delov-Rogoza and my previous experience in math has been somewhat of an unpleasant one. I wouldn’t say that I hate math, there have just been points in my academic career where I have struggled with certain concepts and there have also been times where I have excelled. To sum up my Math experience I would say that my proficiency in math has been a roller coaster ride. Looking back on it, I can say that my struggles in math were not because I was lazy or uninterested. At times, my struggles were a result of the lack of availability for extra help and I also believe that I would have benefit more from the constructivist approach teachers are taking to teach math today.

            I do not want to put the blame on the teachers or the education system but sometimes students are unfortunately put in classes with over thirty students and there is not much of chance for teachers to help those that may require some additional support. Tutoring is an option but for some it may be too expensive to pay for math assistance. Therefore, I believe that it is imperative that schools have extra support services for students that may need additional assistance or that little push to get them in the right direction. Over the years my proficiency in math has slowly progressed with practice, patience, and confidence.

After the first class, I feel a lot more confident about teaching math. We learned that there have been changes to the Ontario Curriculum and students are now being educated with more of a cooperative approach in educating them and providing them with open-ended math questions so that students are able to cooperatively work on the problems. This will stimulate the student’s minds because they are going to ask questions and want to inquire to get a better understanding of the particular problem. It provides students with differentiated methods of learning so they are able actually understand the processes and steps to figure out a math problem and not just simple input a number into a calculator like the old way that students were taught.

Teachers are now teaching students to have a growth mindset and I believe that this is extremely important for the success of many young minds. It used to be okay for students to have fixed mindsets when it came to math. We would often hear many students say “ I’m not good at math” or “I hate math” and this would sometimes be openly accept by teachers, suggesting that maybe this student isn’t good at math? I would argue that as a teacher, I am responsible to install a growth mindset into my students. Suggesting that their abilities can be developed and improved upon through dedication and hard work.  I am looking forward to entering the minds of students and helping them develop into individuals with math mindsets!