Teaching strategies

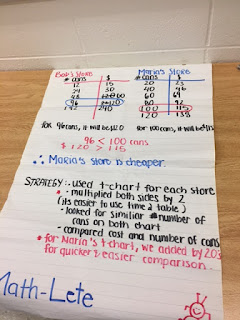
This week in class we participated in a math congress. Mathematical communication and discussion is essential for learning mathematics because as we communicate we are able to reflect on, clarify, and expand our ideas. The math congress was a great exercise that began with the teacher assigning students to work in a group and work together on chat paper to create a solution to the problem that the teacher assigns. The poster should have ideas that are well thought and should present important strategies and concepts that were used to solve their problems. While the students are working away on their problems, it is a great time for the teacher to walk around the class and assess how the students are doing. In our Math congress in class Pat was walking around as we were working on our problems and she got a great idea of how everyone was doing in regard to their understanding of the problem and she was able to see the different mathematical strategies and the different ideas that each of our groups were working towards. I can definitely see myself using a math congress in my classroom because I think that it is extremely important to have discussions in math and if students are not comfortable discussing mathematics with the teacher during the lesson, this gives them the opportunity to discuss ideas and concepts with their peers and gives them the chance to collaborate critically.

As we finished up working on Joel’s Kitchen problem, we posted our solutions around the class and has a gallery walk. This is a unique strategy that has studnets move from station to station to view everyone else’s work. the gallery walk allows students to get into discussion about the problem in a mode of active engagement. It allowed us to see the many different strategies and methods that others took, which helped us gain a deeper understanding of Joel’s problem. I like this strategy a lot because it helps the students understand that there is more than one way to solve mathematical problems and this is beneficial because some individuals may not get how to do a mathematical problem one way, but they understand how to do it another. The gallery walk is also beneficial to teachers because it allows the teacher to monitor the classroom and assess how the students are collaboratively working to understand the mathematical concepts.

After the gallery walk, it is a good strategy to have students return to their original groups to discuss things that they noticed and to reflect on the overall process. Students can then decide if they would like to add things to their original problem and think about whether or not they would have approached the problem in the same way, with their newly acquired information. This gives students opportunities to come to final conclusion on what they saw and discussed and it also gives the teacher the chance to assess the overall classroom’s understanding of the problem and allows the teacher to provide feedback.

Satisfaction With Fractions Pt. 2

   We are approaching week 6 in Math class and this week we finished up working with fractions using division. We began the class with an exercise of Red Light, Green Light. This game had students engage in the activity where the goal of the game is to get from one end of the playing area to the other, as the teacher says “red light” to stop and “green light” to go. Afterwards, we worked with a problem that had examples of the student’s final placement in the game, which was represented by a fraction. For example: Kevin finished 8/9, Kelly finished 5/6, and Lianne ¾, etc. Students were then asked to organize the fractions in order. This activity was great because it was fun and interactive. It allowed students to participate, regardless of their math comprehension level and there were many different ways that students could approach this problem. As teachers, we must teach our students the big ideas about fractions. We have provide students with the basic techniques so that they are able to recognize what a fraction is. A fraction is formed by splitting a whole into any number of pieces of equal size. They should be able to recognize that factions and ratios are the same and that whole numbers are not fractions. A big part of learning fractions is recognizing and differentiating “what is” and “what is not” considered a fraction. Once students have these foundations, it makes it much easier for them to begin adding, subtracting, multiplying, and dividing fractions.

 In class we also had a word problem that revolved around a story where a man named Mr .Tan had a very important porcelain artifact. He accidentally dropped this artifact and it broke in seven pieces. He noticed that the pieces had broken in different shapes and sizes and wonder if he could put this artifact back together. As a class, we were given the task to help Mr. Tan put his artifact back together using similarly shaped tangram pieces. We had to find a way to put our seven pieces back together in a square as displayed in the image below. Then as a class we tried to estimate what faction of each shape, fit into the square.

 I really enjoyed this activity and thought that it was a great way for students to get involved with thinking about fractions. When I was a student I would have really appreciated working on a word problem like this because it was something that was fun, interactive, and included a mixture of storytelling and hands-on activity, where everyone can get involved. Also, working with tangrams is always appealing to students because they are fun and they allow students to visually see the size of the shapes, so that they can explore many different ways to repair their square artifact. Even if the student cannot repair their square using the tangrams, they can still see the size of the shapes and this could help them determine the potential fraction that each shape represents to make the entire square.

Satisfaction With Fractions

We have just finished week 4 in math class and things are still going very well. My practicum has given me the opportunity to see how students math proficiency is assesses, as the students in my practicum class have had math assessments on both days of my placement. This has been a good experience thus far because I have been able to look at some of the student’s tests and see how the teacher grades the assessment, according to the marking rubric. It has also given me the opportunity to see some of the different ways that students approach particular math questions. I found it very interesting that some students are very particular with showing their work and other do not do this but they still get the answers correct.  I struggle with this because I think it leaves teachers in a dilemma. If a student consistently gets his or her answers right but doesn’t show their work, do we mark it wrong? Do we give part marks? I know that showing your work is an extremely important thing to do in math but not every student will do this. We unfortunately do not live in a perfect world. So, do we suggest that if the student doesn’t show their work, they do not understand the material or the expectations? Is the student just lazy? That is some food for thought.

The only downside to my practicum thus far, is that I haven’t had the opportunity to see how my practicum teacher teaches the math material. Hopefully when we are back in our observation period I will have the opportunity to see my practicum teacher’s instructional methods before I begin my full-time placement in her class. Thankfully, our math class has been very informative and highly beneficial thus far, so I feel more confident about teaching math.

I really enjoyed our last class. We read the Hershey’s book on fractions. I think that this is an excellent resource for teachers to help their students work with and understand fractions. Having chocolate bars as a manipulative to work with fractions is genius. Students get to see visual representations so that they can understand how fractions work and get the chance to work through it with their peers and demonstrated their understanding. We also used other manipulatives such as the ones shown below, to work with fractions and see how many different manipulatives can be used to help students gain a deeper understanding of how to work with various types of fractions. The egg carton was another strategy used as a visual representation to increase students understanding on how fractions work. As we add and remove eggs to the carton, students can figure out that 6 eggs in a carton of 12 equals ½, ect.

 As teachers, it is important for us to give students visual representations and come up with questions that are open-ended. This gives the students the opportunity to engage in discussion about the problem with their peers and while this is happening, the teachers can assess the students understanding of the problems and provide them with feedback.